Binary hands

I think no one will question the fact that the age of nine is a beautiful stage of life. But if somebody thinks a person of this age – being a thinking person without any doubts –  is the impersonation of gravity, steady and predictable, then… they probably forgot how it was. The vivid mind is one thing, and the body, constantly demanding its share of exercise is …

 … well, that’s it! We needed just a few bricks for the class, and now the entire content of the container lies in front of us in a pile and I have to confront several pairs of pleading eyes, staring at me.

‘Shall we count them, pleeease?’

 I am always amazed by the passion and, at the same time, the patience, with which children are prepared to count large sets of identical items, join bricks in giant towers of Babel, as if they wanted to catch the numbers red-handed, making sure for the hundredth time if this one isn’t the largest number in the world, which, allegedly, doesn’t exist. Refusal would be sheer cruelty. With the prospect of the next our spent happily moving bricks from one pile to another, I desperately search my mind for the way of accelerating this process. Theoretically, I have several pairs of laborious, eager-to-help hands at my disposal… but how to use them? Everybody does their own counting and then the results are totaled? – Hmmm, looks like a screenplay of the “War for the bricks” movie ready to put into practice. In tens? – Awkward, boring and the chance of a mistake pretty high, while the young hunters for the truth want to know the TRUE number of the bricks. And, last but not least, the “war for the bricks” doesn’t look much less likely.

Wait, wait… I think I have got it! Not in tens, but in twos, yes! And then the twos again in twos, and so on. With so many  helpers it should go pretty fast, virtually without a risk of a mistake, and… practically without counting. Off we go!

 The pile of bricks is melting away quickly.

In less than two minutes the pairs are already dominating and this is the longest stage – the rest should go in no time.

Done! Not a single brick has remained, so we can now start pairing the pairs.

As predicted, it goes even faster.

Finished! This time there is one odd pair out, so we are moving it to the side.

The created sets are being paired again.

This time no single set has remained, so we can continue pairing still larger sets of bricks. The “remainders” start forming the binary notation of our number, ordered from the back to the front. The zeros indicate that neither a single brick, nor a single “four” was left.

At the next stage each group was paired again, so another zero appears in the sequence.

The same is repeated in the subsequent step and another zero is added.

This time one set remains unmatched...

 --- and a „one” is added to our binary sequence.

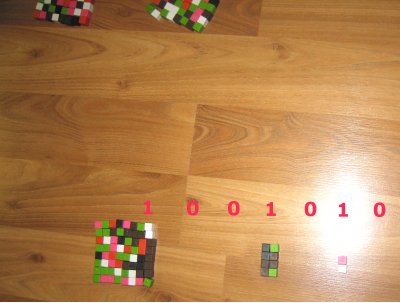
We are nearly there. The remaining sets become pretty large, while their number is dwindling rapidly. Will any of them find its pair this time?

Yes! Another zero appears before the „one” and we are left with only two sets, which will also create a pair without a remainder, bringing another zero to our number. The last one, actually, because this last joint set will of course remain single, represented by a “one” at the beginning of the sequence.

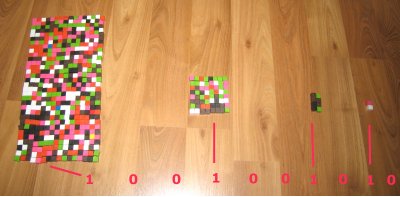
Finished! We did it within ten minutes, doing together an instructive activity and getting an answer to a question about the number of the bricks in the container, practically without the risk of a mistake.

And this answer iiiiiis… Come on, who will give me the total number of the bricks?